Motion and Force

Unit: 7

Book Icon Class 10: Science

Gravitational Force, Newton’s Law of Gravitation, Gravity, Acceleration Due to Gravity, Coin and Feather Experiment, Height and Acceleration Due to Gravity, Mass and Weight, Freefall, Weightlessness, Equation of Motion for Freefall

Gravitational Force

The attraction force between two celestial bodies is called gravitational force. Its SI unit is Newton(N).

Newton’s Universal Law of Gravitation

Newton’s Law of Gravitation states that,

“The gravitational force between two celestial bodies is proportional to the product of their masses and inversely proportional to the square of their central distance.”

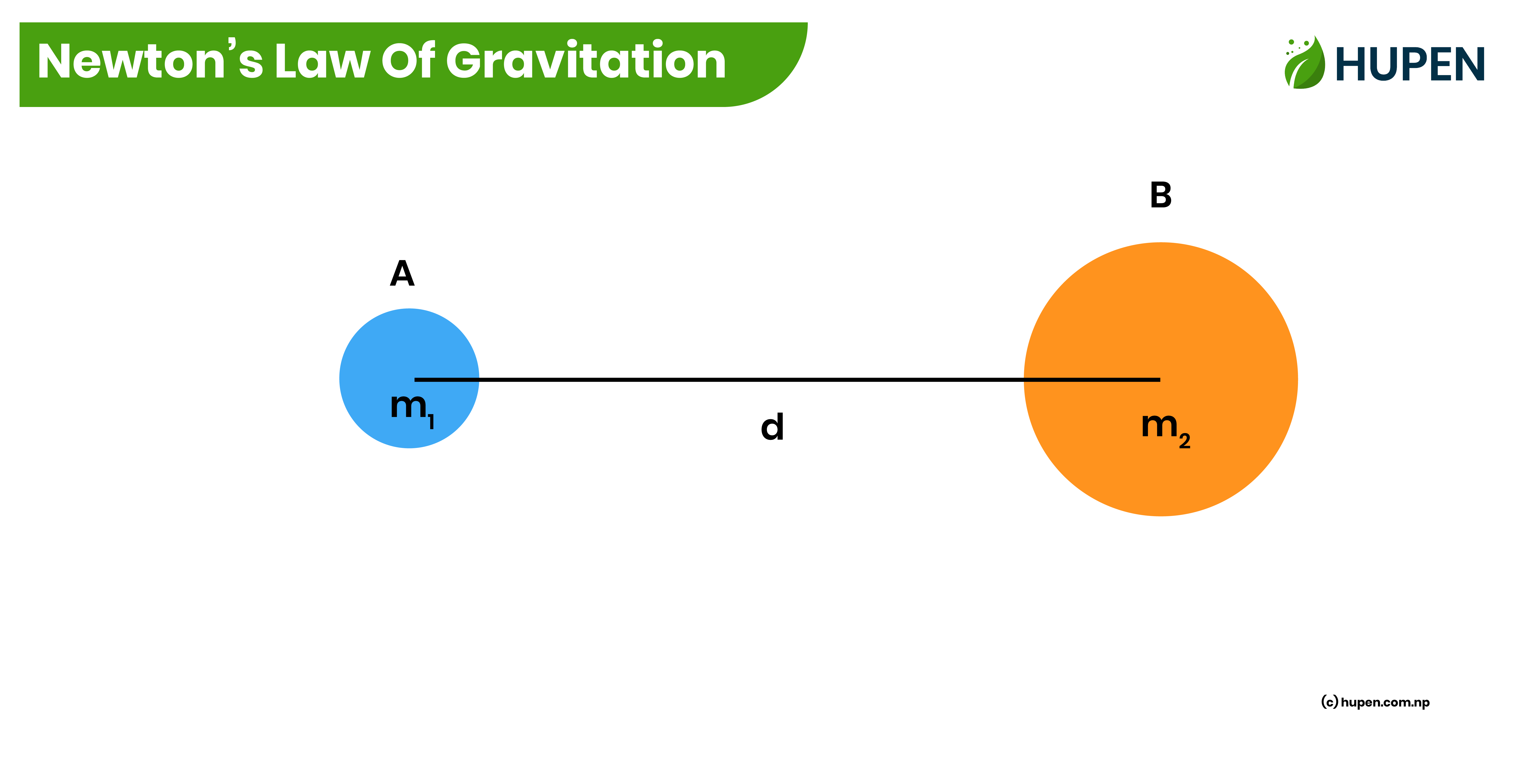
Let us consider the two bodies A and B having masses m₁ and m₂ respectively are at distance d. Then, if the gravitational force is F between them then,

According to Newton’s law of gravitation,

\( \begin{aligned} & F \propto m_1 m_2 \quad \text{------(i)} \\ & \text{and,} \\ & F \propto \frac{1}{d^2} \quad \text{------(ii)} \\ \\ & \text{Now, from equations (i) and (ii)} \\ \\ & or, F \propto \frac{m_1 m_2}{d^2} \\ \\ & \therefore F = G \frac{m_1 m_2}{d^2} \\ \\ & \text{Where, G is a proportionality constant called Gravitational Constant.} \\ & \text{Which is the required equation.} \end{aligned} \)

Gravitational Constant (G)

The force of attraction between two bodies having a mass of 1 kg each and separated by a distance of 1 m is called the gravitational constant. Its value is equal to 6.67 x 10^-11 Nm²/kg². Henry Cavendish calculated its value by using a sensitive balance called the Torsion balance in 1978 AD.

Variation In Gravitational Force with Mass & Distance

Let us consider the two bodies A and B having masses m₁ and m₂ respectively are at distance d. Then, if the initial gravitational force is F between them then,

According to Newton’s law of gravitation,

\(F = G \frac{m_1 m_2}{d^2} \quad ....... (i)\)

1. Keeping the distance constant while varying the masses.

When the mass of an object is doubled	When the masses of both objects are doubled
\( \begin{aligned} & \text{putting } m_1 = 2m_1 \text{ in eqn (i) } \\ & or, F_2 = G \frac{2m_1 m_2}{d^2} \\ \\ & or, F_2 = 2G \frac{m_1 m_2}{d^2} \\ \\ & \therefore F_2 = 2F \end{aligned} \)	\( \begin{aligned} & \text{putting } m_1 = 2m_1 \text{ and } m_2 = 2m_2 \text{ in eqn (i) } \\ & or, F_2 = G \frac{2m_1 2m_2}{d^2} \\ \\ & or, F_2 = 4G \frac{m_1 m_2}{d^2} \\ \\ & \therefore F_2 = 4F \end{aligned} \)

Conclusion: If the mass of an object is increased by two times, the gravitational force increases by two times. Also, if the masses of both objects are increased by two times, the gravitational force increases by four times.

Keeping the masses constant while varying the distance.

When the distance is reduced by half	When the distance is increased by two times
\( \begin{aligned} & \text{putting } d = \frac{d}{2} \text{ in eqn (i) } \\ & or, F_2 = G \frac{m_1 m_2}{(\frac{d}{2})^2} \\ \\ & or, F_2 = 4G \frac{m_1 m_2}{d^2} \\ \\ & \therefore F_2 = 4F \end{aligned} \)	\( \begin{aligned} & \text{putting d = 2d in eqn (i) } \\ & or, F_2 = G \frac{m_1 m_2}{(2d)^2} \\ \\ & or, F_2 = \frac{1}{4} G \frac{m_1 m_2}{d^2} \\ \\ & \therefore F_2 = \frac{F}{4} \end{aligned} \)

Conclusion: If the distance is decreased to half, the gravitational force increases by four times. Also, If the distance is increased by two times, the gravitational force decreases by four times.

Consequences Of Gravitational Force

The gravitational force exerted by the sun and moon gives rise to the tides experienced in seas and oceans.
The sun acts as the center of attraction around which planets orbit.
The presence of solar systems and galaxies is a direct consequence of gravitational force.
Gravitational force governs the orbital motion of planets, moons, and satellites around larger celestial bodies.
The gravitational force of the Earth enables rainfall and snowfall to occur.

Gravity

The force of attraction with which a planet, satellite or star pulls a body towards its center is called gravity. Its SI unit is Newton(N). All planets and heavenly bodies have their own gravity but the magnitude of force of gravity depends upon mass and radius.

Effects of Gravity

Acceleration is produced on freely falling body.
Every object has some weight.
The earth is surrounded by the atmosphere.
All objects fall towards the surface from a certain height.

Acceleration Due to Gravity

The acceleration produced in a freely falling body due to the force of gravity of a planet is called acceleration due to gravity. It is denoted by ‘g’. Its SI unit is N/m².

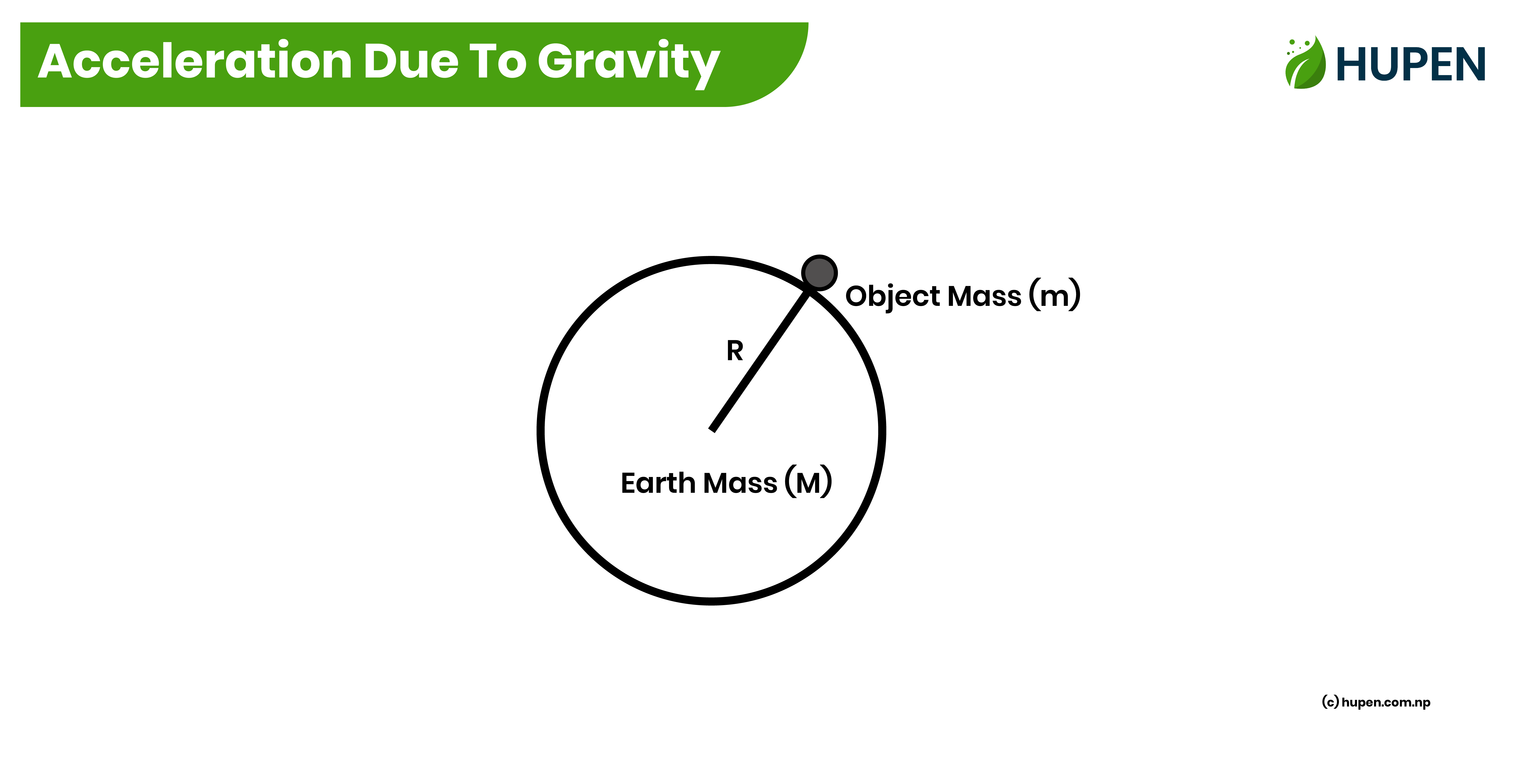
Let the mass of any planet or satellite be M, its radius R and mass of an object m, then according to Newton’s universal law of gravitation,

\( \begin{aligned} & F = G\frac{Mm}{R^2} \quad ...... (i) \\ & \text{Also, According to Newton's Second Law,} \\ & F = mg \quad ...... (ii) \\ & \text{From, eqn (i) and (ii)} \\ \\ & or, mg = G\frac{M m}{R^2}\\ \\ & \therefore g = \frac{GM}{R^2} \\ & \text{Which is the required expression.} \end{aligned} \)

In above equation G is universal gravitational constant and M is the mass of the planet. Therefore, both are constant. So, we can write,

\(\therefore g \propto \frac{1}{R^2}\)

Therefore, it makes clear that the mass of an object does not affect the acceleration due to gravity in falling objects. Thus, the acceleration due to gravity is inversely proportional to the square of the planet’s radius.

Variation In Acceleration Due to Gravity

The Earth is an oblate spheroid, meaning it is mostly spherical but slightly flattened at the poles and bulging at the equator. As a result, the acceleration due to gravity is slightly higher at the poles, measuring approximately 9.83 m/s², compared to around 9.87 m/s² at the equator. To simplify calculations, an average value of 9.8 m/s² is commonly used to represent the acceleration due to gravity on Earth.

Coin And Feather Experiment

The feather and coin experiment demonstrates the impact of air resistance on falling objects. In a vacuum, both objects fall simultaneously and at the same rate, highlighting the absence of air resistance. In normal conditions, the feather falls slower due to air resistance, while the coin falls faster. This experiment emphasizes the influence of air resistance on falling speeds and the equal acceleration of objects in a vacuum under gravity.

Conclusion: The acceleration produced due to gravity is same in all object if there is no air resistance.

Height And Acceleration Due to Gravity

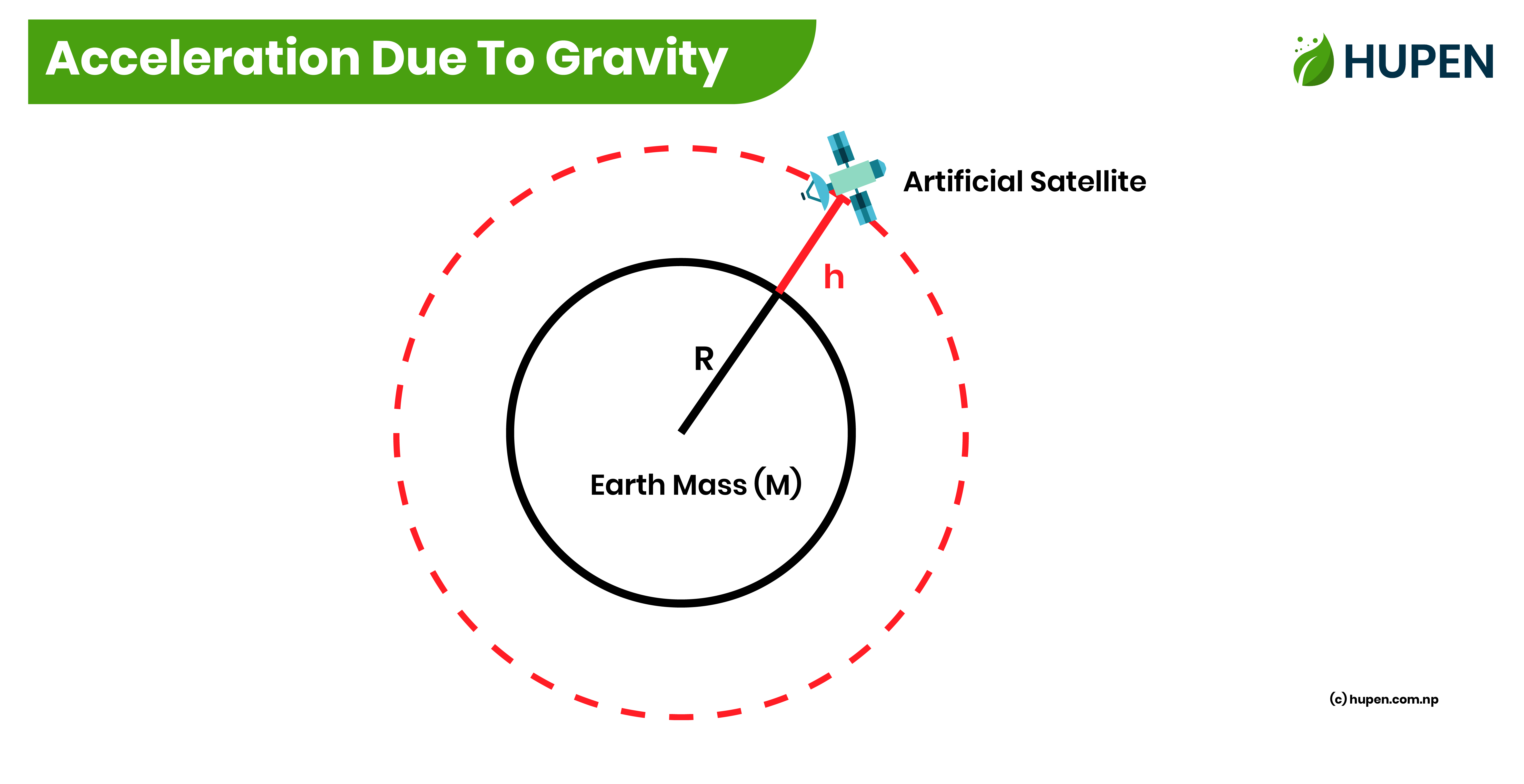
The acceleration due to gravity at height ‘h’ from the surface is calculated as,

\( \begin{aligned} F = \frac{GM}{(R+h)^2} \end{aligned} \)

Mass And Weight

The total quantity of matter contained in a body is called its mass. The SI unit of the mass is Kg. It is measured by a physical or a beam balance.
The force with which earth attracts any object on its surface towards its center is called weight. Its SI unit is Newton(N).It is measured by spring balance.

The weight of an object is calculated as, W = m x g

Where, m = mass of the body, g = acceleration due to gravity

Freefall

An object falling without any resistance is called Freefall. The acceleration produced on an object during the freefall is equal to the acceleration due to gravity at that place. There is no perfect freefall of the object falling on the earth due to air resistance. The surface of the moon is not covered by the air. So, the falling of any object on moon is freefall.

Weightlessness

The weight of an object falling freely under the effect of gravity appears to be zero. It is called Weightlessness.

Conditions for weightless

When a body is falling freely under the action of gravity, it becomes weightless.
When a body is in the space or at null point, it becomes weightless.
When a body is in the center of the earth.
Inside an artificial Satellite revolving around the earth or heavenly body.

Equation Of Motion For Free Fall

For equation of motion for free fall, we have to modify the equations of motion in straight line. For that we have to replace ‘S’ with ‘h’ and ‘a’ with ‘g’.

Equation of Motion	Equation of Motion in Free Fall
\( v = u + at \)	\( v = u + gt \)
\( v^2 = u^2 + 2as \)	\( v^2 = u^2 + 2gh \)
\( s = ut + \frac{1}{2} at^2 \)	\( h = ut + \frac{1}{2} gt^2 \)

LESSON CONTENTS

Share Now

Feedback Report an Issue

Lesson Completed successfully.