Circle, Central Angle and Inscribed Angle, Relation Between Central, Inscribed Angle and its corresponding Arc, Relation between central angle and ins...
AI-Powered
TL;DR — Quick Summary
Click Generate Summary to get a quick AI-powered overview of this chapter.
A circle is a 2D geometrical shape defined as the set of all points in a plane that are equidistant from a given point called the center.
Here are the key definitions related to the parts of a circle:
1. Radius (r): The distance from the center of the circle to any point on its circumference.
2. Diameter (d): A straight line passing through the center, connecting two points on the circumference; it is twice the radius. This is the longest chord in a circle.
3. Circumference: The total length around the circle, calculated as \(2\pi r\) or \(\pi d\).
4. Area of Circle: The space inside the circle, given by \(\pi r^2\).
5. Center: The exact midpoint inside the circle.
6. Chord: A straight line connecting two points on the circumference. It can vary in length from zero (if it's just a point) to the diameter.
7. Arcs: Segments of the circumference between two points. They can be minor arcs (less than 180 degrees) or major arcs (more than 180 degrees).
Additionally:
8. Tangent Line: A straight line touching the circle at exactly one point and not crossing through its interior.
Cyclic Quadrilateral
A cyclic quadrilateral is a quadrilateral whose all four vertices lie on the circumference of a single circle. This means that it can be inscribed inside a circle. Hence, a quadrilateral inside a circle with four vertices on its circumference.
Properties of a Cyclic Quadrilateral:
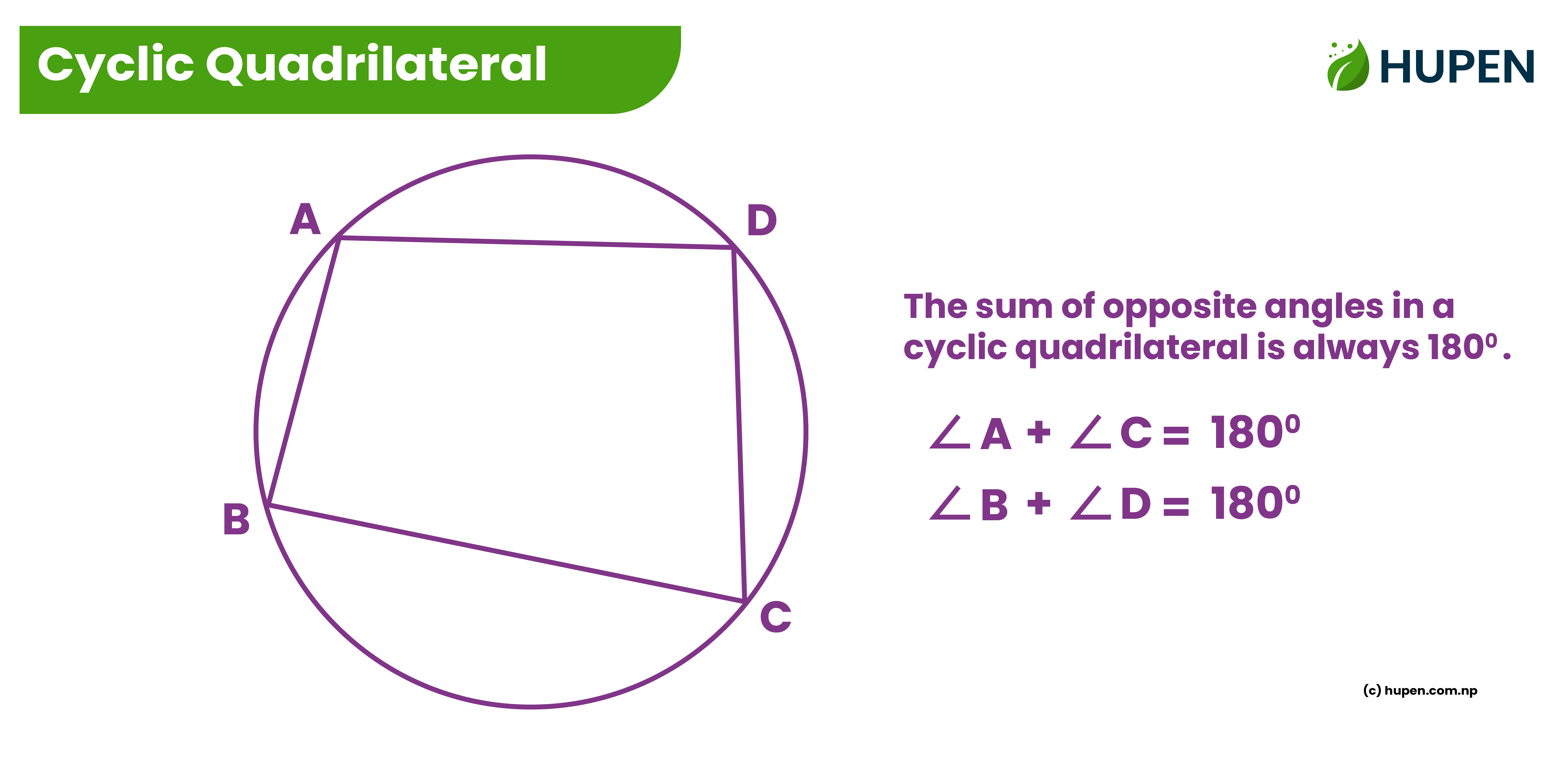
1. Opposite Angles are Supplementary:
- The sum of opposite angles in a cyclic quadrilateral is always \(180^\circ\).
\(\angle A + \angle C = 180^\circ, \quad \angle B + \angle D = 180^\circ\)
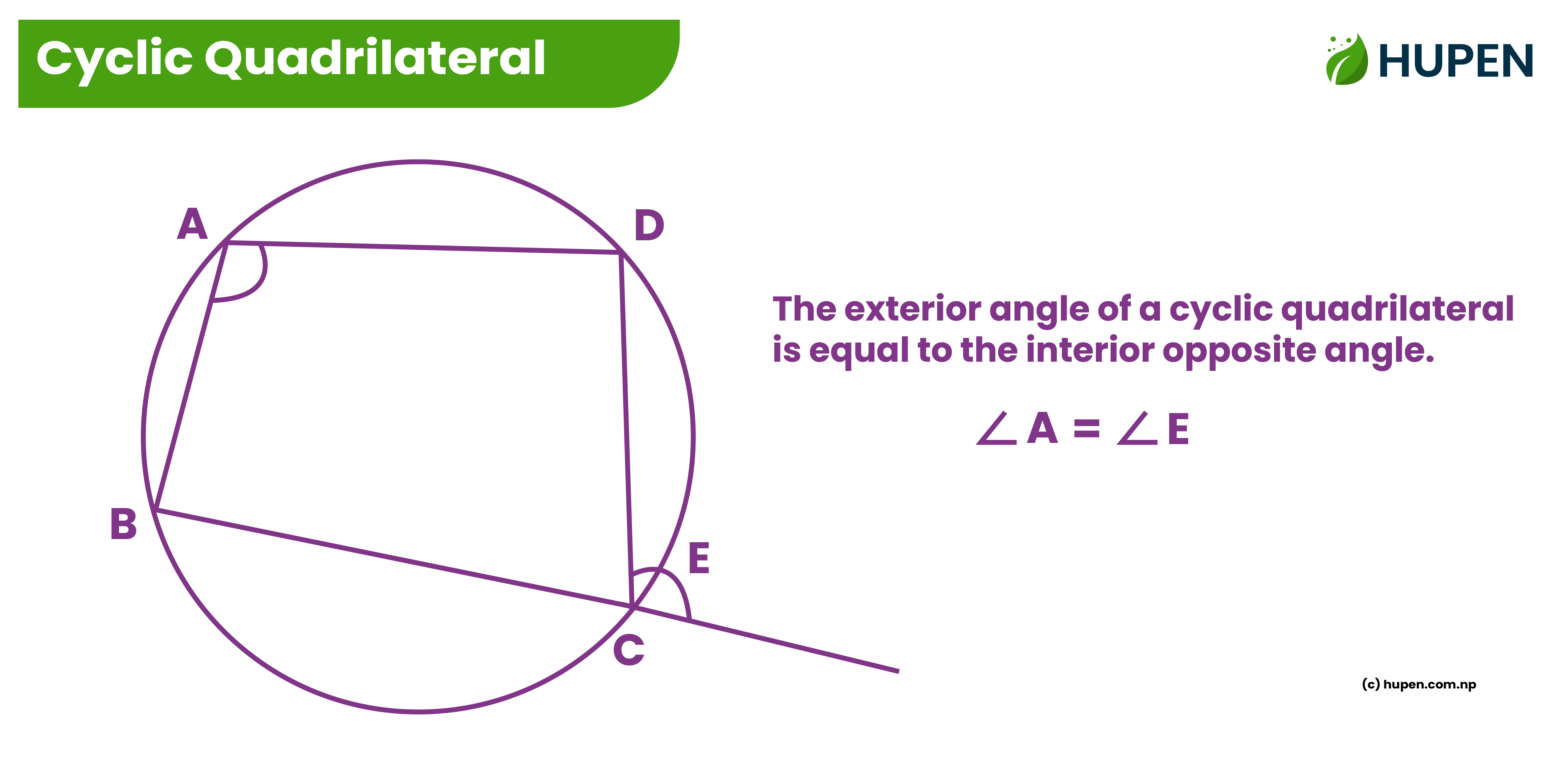
2. Exterior Angle Property:
- The exterior angle of a cyclic quadrilateral is equal to the interior opposite angle.
\(\angle E = \angle A\)
Practice Exercises
Reinforce your learning! Attempt these exercises to
build deep mastery and prepare for your quizzes.
Q1.
In a circle there are four points M, U, S and A at the circumference. If \(\angle MUA\) and \(\angle MSA\) are the angle at the circumference. [SEE 2080 LP]
a.Write the relation between \(\angle MUA\) and \(\angle MSA\). [1K]
b.If \(\angle MUA = 6x + 10^0\) and \(\angle MSA = 7x – 10^0\) then find the value of \(\angle MUA\). [1U]
c.Verify experimentally that the angles at the circumference standing on the same arc of a circle are equal. (Two circles of radii not less than 3 cm are necessary.) [2A]
